To facilitate our proofs in what follows, some equilibrium concepts of the dynamic are in order. Say that a state
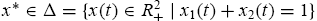is a dynamic equilibrium (a.k.a. steady state) for the dynamic
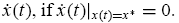Such states are steady in that
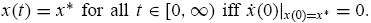
Say that a dynamic equilibrium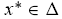 
is asymptotically stable if it has some open neighborhood N(x¤) such that limt!1
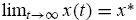, if the initial state
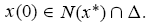Intuitively, the asymptotic stability requires a local pull toward the steady state following a small perturbation, and hence all states near a dynamic equilibrium will eventually evolve toward it.
Proof of theorem 1. Note that the homogenous profiles (1, 0) and (0, 1)
are always steady states for the dynamic in (18) and the heterogeneous profile
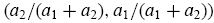is an interior steady state if the two return parameters, a1 and a2, have the same signs. To check the asymptotic stability, differentiate the dynamic ˙x2 in (18) with respect to x2 and evaluate the partial derivative at each steady state, respectively, as follows:
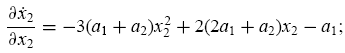
(A1)
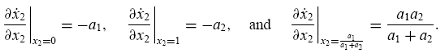
(A2)
Asteady state is asymptotically stable iff the partial derivative evaluated at that state is negative. By (A2), the following results are obtained: (1, 0) is asymptotically stable if a1 > 0; (0, 1) is asymptotically stable
if a2> 0; (a2/(a1 + a2), a1/(a1 + a2))
is asymptotically stable if a1< 0 and a2< 0. j
The results of the proof of theorem 1 are summarized as follows:
(I) If a1> 0 and a2< 0, then (X1; X2) = (1,0) is the unique asymptotically
stable equilibrium.
(II) If a1< 0 and a2> 0, then (X1, X2) = (0,1) is the unique asymptotically
stable equilibrium.
(III) If a1> 0 and a2> 0, then there exist two asymptotically stable equilibria such that
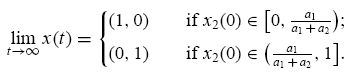
(VI) If a1< 0 and a2< 0, then (X1,X 2) = (a2/(a1 + a2), a1/(a1 + a2)) is the
unique asymptotically stable equilibrium.
Proof of theorem 2.1. By (30), if
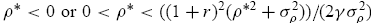
then we have
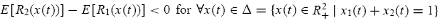
To derive the parameter space for the second case, rearrange
and write a quadratic function ƒ (P¤) as follows:
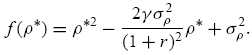
A simple calculation shows that the function is greater than zero (and hence
if either
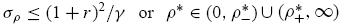where
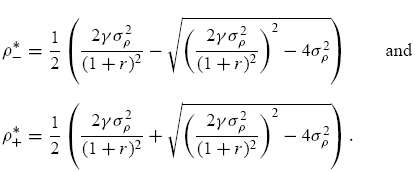
Given that E[R2(x(t))]-E[R1(x(t))] < 0, starting from any nonstationary states, the population share of noise traders will decline to zero in the long run. Thus, the unique asymptotically stable equilibrium is (X1, X2) = (1,0).
On the other hand, if
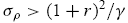and
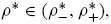then the function ƒ (P¤) is less than zero, and hence the dynamic in (30) may have one interior
steady state, (X1, X2) = (1-µ,µ), where µis given by
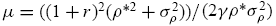
In this case, the expected return differential, E[R2(x(t))] - E[R1(x(t))],
depends on the current population share relative to the interior steady state. If the current noise trader share is below the interior steady state, i.e., X2(t) < µ, then the expected return differential is negative and the population share of the noise traders will decline to zero in the long run. If the current noise trader share is above the interior steady state, i.e., X2(t) > µ, then the expected return differential is positive and the population share of the noise traders will increase to one. Thus, we obtain two asymptotically stable equilibria, (1,0) and (0,1), respectively.
The results of the proof of theorem 2.1 are summarized as follows:
(I) If P¤ < 0, then (X1, X2) =(1,0) is the unique asymptotically stable equilibrium.
(II) If
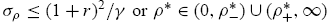then (X1, X2) = (1,0) is the unique asymptotically stable equilibrium.
(III) If
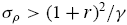and
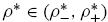then there exist one unique interior
steady state (1 - µ,µ) and two asymptotically stable equilibria such that
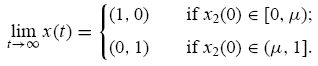
Proof of theorem 2.2. By (29)
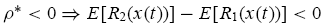for
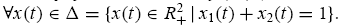
Hence, the dynamic in (29) only has two steady states: (1, 0) and (0, 1). To check the asymptotic stability, differentiate the dynamic ˙X2 in (29) with respect to X2 and evaluate the partial derivative at each
steady state, respectively, as follows (ignoring the constant 1/c0 without loss of generality):
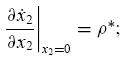
(A5)
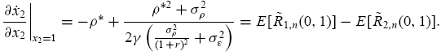
Asteady state is asymptotically stable iff the partial derivative evaluated at that state is negative. Hence x(t) =(1,0) is the unique asymptotically stable equilibrium in category I in which P¤ < 0. In category II (i.e., P¤ > 0 and E[R2(x(t))] ¡ E[R1(x(t))] > 0 for
the dynamic again has two steady states: (1, 0) and (0, 1). Given that P¤ > 0; x(t)= (1,0) is not asymptotically stable by (A5). On the other hand, (0, 1) is asymptotically stable iff
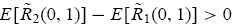by (A6). This always holds in category II by its definition. Hence, x(t) D (0; 1) is the unique asymptotically stable equilibrium.
If P¤ > 0 and g > 0, then
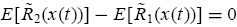
may have at most two real roots µL and µH as defined in (31) and (32), for some
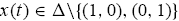
By definition, a positive real root is an interior steady state if it is bounded above by one. Hence, there are two possibilities for the existence of the interior states: (1) the lower root µL is the unique interior steady state in category III for 0 < µL< 1 < µH, and (2) both roots µL and µH are interior steady states in category IV for 0 < µL< µH< 1. To check the asymptotic stability for the interior steady states, compute
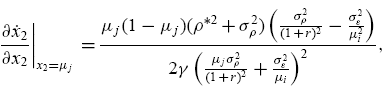
where j = L, H:
Equation (A7) indicates that an interior steady state µj is negative iff
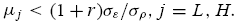Given the existence of the two real roots, i.e., g > 0, (31) and (32) imply the following two inequalities:
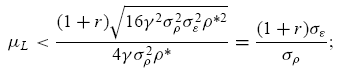
(A8)
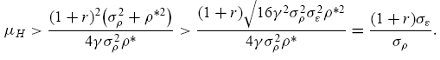
(A9)
By (A7)–(A9), the lower root µL is asymptotically stable, but the higher root µH is not. Note that (0,1) is asymptotically stable iff
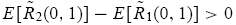
by (A6). Note also that
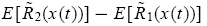is a convex function, given
P¤ > 0. Thus, a simple inspection of the convex function for
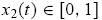shows that (1)
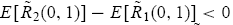if there exists only one interior steady state µL (category III) and (2)
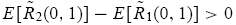
if there exist two interior steady states (category IV). Hence, x(t) D (0; 1) is an asymptotically stable equilibrium in category IV, but not in category III. Finally, given that P¤ > 0; x(t) = (1,0) is not asymptotically stable in either category III or IV by (A5). Therefore, we obtain the desired results in Theorem 2.
The results of the proof of theorem 2.2 are summarized as follows:
(I) If P¤ < 0, then (X1,X2) = (1,0) is the unique asymptotically stable equilibrium.
(II) If P¤ > 0 and
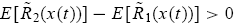for
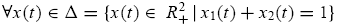
then (X1,X2) = (1,0) is the unique asymptotically stable
equilibrium.
(III) If P¤ > 0 and 0 < µL< 1 < µH; then (X1,X2)=(1 - µL,µH) is the
unique asymptotically stable equilibrium.
(IV) If P¤> 0 and 0 < µL< µH< 1, then there exist two asymptotically
stable equilibria such that
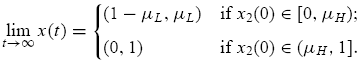
Prof. F. Albert Wang
Next: References
Summary: Index