Let f be a smooth dynamical system defined on an n-dimensional compact manifold

M:

^s(t) = f t(8(0))

where s(t) is the state of the system at time t. The map f thus takes an initial state s(O) to a state s(t) where the time variable t can either be continuous or discrete. Associate with the dynamical system in eq. (2.1) an observer function h : M - R

which generates the data points in the observed scalar time series:

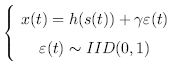(2.2)

where y is the noise level and e(t) is the measurement error. More precisely, assumethat the observer function, sampled at time intervals Ts , generates the N-point timeseries

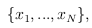(2.3)

Note that observational noise is present in the observed timeseries. For the moment, however, it is assumed that the observed time series isnoise-free, i.e. y = 0 in eq. (2.2).

As mentioned above, the past and the future of an observed scalar time seriescontain information about unobserved state variables that can be used to define a state at the present time. Specifically, let

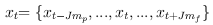
be the reconstructed state at time t where

is the reconstruction delay and,

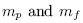
are the number of data points in the time series taken from the pastand the future, respectively. The lag time is

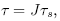
the window length is

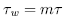
and the dimension of the delay vector in eq. (2.4), i. e. the embedding dimension, is

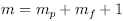
Moreover, the number of states on the reconstructed trajectory in the reconstructed phase space are

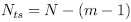
Thus, the reconstructed

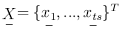
where

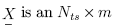
and the superscriptdenotes transpose. For example, assume that the observed time series consists of ten data points, trajectory is i. e.N = 10 in eq. (2.3). Further assume that the reconstruction delay is equal to two, i.e. J = 2 in eq. (2.4), and that Mp = 0 and Mf = 3 in eq. (2.4). This meansthat the reconstructed trajectory can be written as

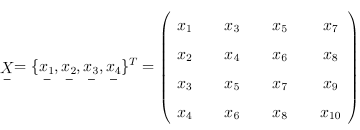
Takens (1981) proved that the delay reconstruction map

(2.5)

which maps the original n-dimensional state s onto M-dimensional delay vectors, isan embedding if

If is an embedding then a smooth function g is inducedon the reconstructed phase space:

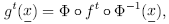(2.6)

where o is the operator for the composition of mappings. The reconstructed phasespace can thus be used to estimate g and because g is topologically conjugate tothe true but unknown dynamical system f in eq. (2.1), i.e. they are "equivalent",g can be used to estimate relevant statistical properties of the dynamics, e.g. the Lyapunov exponents.

An intuitive explanation of Takens' (1981) embedding criterion, i.e. M > 2n, is as follows: Assume that

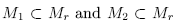
are two subspaces of dimension n1 and n2, respectively, where Mr is an M-dimensional manifold representing thereconstructed phase space. In general, the two subspaces intersect in a subspace ofdimension n+ n2 -M. Thus, if this expression is negative, there is no intersection1 of the two subspaces. Of greater interest, the intersection of two subspaces of thesame dimension, or the self-intersection of an n-dimensional set with itself fails to occur when n + n - m < 0, i. e. when m > 2n.

Sauer et al. (1991) contains several generalizations of Takens' (1981) embedding theorems. For example, for a chaotic attractor

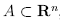
, almost every delay reconstruction map

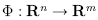
is an embedding on smooth manifolds contained within A provided that m is twice as large as the dimension of A. Broadly speaking, an attractor is a compact set A with the property that there is a neighborhood of A
such that for almost every initial condition, the limit set of the trajectory defined by the dynamical system, i. e. once transients have disappeared, is A. These initial conditions are the basin of attraction of the attractor. The distinction between asymptotic and transient behavior is possible if it is assumed that the dynamical system is dissipative, i.e. if volumes in phase space shrink over time.

Prof. Mikael Bask

Next: Choosing the proper reconstruction parameters

Summary: Index